How To Find Missing Angles Of A Parallelogram

Angles of a Parallelogram

At that place are iv interior angles in a parallelogram and the sum of the interior angles of a parallelogram is always 360°. The opposite angles of a parallelogram are equal and the sequent angles of a parallelogram are supplementary. Let usa read more most the properties of the angles of a parallelogram in detail.

one.	Properties of Angles of a Parallelogram
2.	Theorems Related to Angles of a Parallelogram
3.	FAQs on Angles of a Parallelogram

Properties of Angles of a Parallelogram

A parallelogram is a quadrilateral with equal and parallel opposite sides. There are some special properties of a parallelogram that make it unlike from the other quadrilaterals. Observe the following parallelogram to chronicle to its properties given below:

• The contrary angles of a parallelogram are congruent (equal). Here, ∠A = ∠C; ∠D = ∠B.
• All the angles of a parallelogram add together up to 360°. Here,∠A + ∠B + ∠C + ∠D = 360°.
• All the corresponding consecutive angles are supplementary. Here, ∠A + ∠B = 180°; ∠B + ∠C = 180°; ∠C + ∠D = 180°; ∠D + ∠A = 180°

Theorems Related to Angles of a Parallelogram

The theorems related to the angles of a parallelogram are helpful to solve the issues related to a parallelogram. 2 of the of import theorems are given below:

• The contrary angles of a parallelogram are equal.
• Consecutive angles of a parallelogram are supplementary.

Let us larn about these two special theorems of a parallelogram in particular.

Reverse Angles of a Parallelogram are Equal

Theorem: In a parallelogram, the opposite angles are equal.

Given: ABCD is a parallelogram, with four angles ∠A, ∠B, ∠C, ∠D respectively.

To Prove: ∠A =∠C and ∠B=∠D

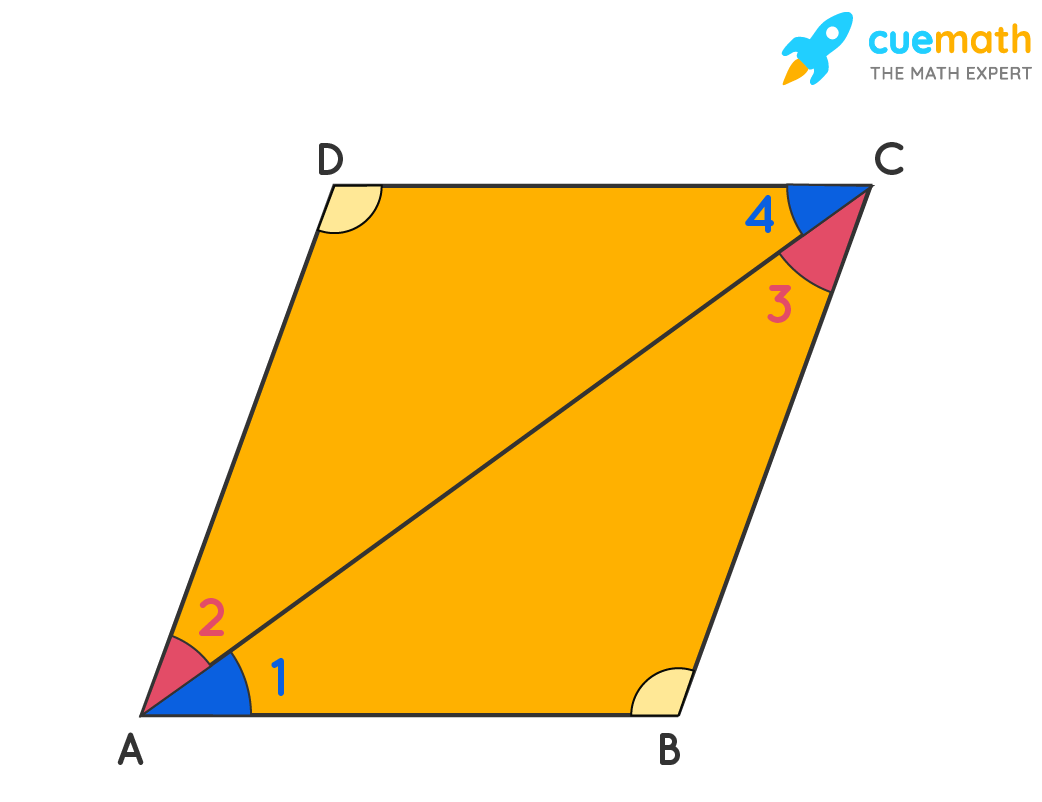
Proof: In the parallelogram ABCD, diagonal Air-conditioning is dividing the parallelogram into two triangles. On comparing triangles ABC, and ADC. Here we have:
AC = Air-conditioning (common sides)
∠1 = ∠iv (alternate interior angles)
∠2 = ∠iii (alternate interior angles)
Thus, the two triangles are coinciding, △ABC ≅ △ADC
This gives ∠B = ∠D past CPCT (corresponding parts of coinciding triangles).
Similarly, nosotros can show that ∠A =∠C.
Hence proved, that contrary angles in whatever parallelogram are equal.

The antipodal of the above theorem says if the opposite angles of a quadrilateral are equal, then information technology is a parallelogram. Let united states of america prove the same.

Given: ∠A =∠C and ∠B=∠D in the quadrilateral ABCD.
To Prove: ABCD is a parallelogram.
Proof:
The sum of all the 4 angles of this quadrilateral is equal to 360°.
= [∠A + ∠B + ∠C + ∠D = 360º]
= 2(∠A + ∠B) = 360º (We tin can substitute ∠C with ∠A and ∠D with ∠B since information technology is given that ∠A =∠C and ∠B =∠D)
= ∠A + ∠B = 180º . This shows that the consecutive angles are supplementary. Hence, information technology ways that AD || BC. Similarly, we can evidence that AB || CD.
Hence, Advert || BC, and AB || CD.
Therefore ABCD is a parallelogram.

Consecutive Angles of a Parallelogram are Supplementary

The consecutive angles of a parallelogram are supplementary. Permit united states prove this belongings considering the following given fact and using the aforementioned figure.

Given: ABCD is a parallelogram, with 4 angles ∠A, ∠B, ∠C, ∠D respectively.
To evidence: ∠A + ∠B = 180°, ∠C + ∠D = 180°.
Proof: If AD is considered to be a transversal and AB || CD.
Co-ordinate to the property of transversal, we know that the interior angles on the same side of a transversal are supplementary.
Therefore, ∠A + ∠D = 180°.
Similarly,
∠B + ∠C = 180°
∠C + ∠D = 180°
∠A + ∠B = 180°
Therefore, the sum of the respective two adjacent angles of a parallelogram is equal to 180°.
Hence, it is proved that the consecutive angles of a parallelogram are supplementary.

Related Manufactures on Angles of a Parallelogram

Check out the interesting manufactures given beneath that are related to the angles of a parallelogram.

• Perimeter of Parallelogram
• Parallelogram Worksheets
• Parallelogram Formula
• Properties of Parallelograms

Solved Examples on Angles of a Parallelogram

1. Example 1: One bending of a parallelogram measures 75°. Observe the measure of its side by side bending and the mensurate of all the remaining angles of the parallelogram.

   Solution:

   Given that one angle of a parallelogram = 75°
   Allow the adjacent angle be x
   We know that the consecutive (adjacent) angles of a parallelogram are supplementary.
   Therefore, 75° + x° = 180°
   ten = 180° - 75° = 105°
   To find the measure of all the four angles of a parallelogram we know that the opposite angles of a parallelogram are coinciding.
   Hence, ∠i = 75°, ∠2 = 105°, ∠3 = 75°, ∠four = 105°

2. Instance 2: The values of the reverse angles of a parallelogram are given as follows: ∠ane = 75°, ∠three = (ten + thirty)°, find the value of 10.
   Given: ∠1 and ∠3 are opposite angles of a parallelogram.

   Solution:

   Given: ∠i = 75° and ∠three = (ten + thirty)°
   We know that the opposite angles of a parallelogram are equal.
   Therefore,
   (ten + 30)° = 75°
   ten = 75° - 30°
   10 = 45°
   Hence, the value of x is 45°.

go to slidego to slide

Breakdown tough concepts through simple visuals.

Math will no longer be a tough field of study, particularly when you understand the concepts through visualizations.

Book a Gratis Trial Class

Practice Questions on Angles of a Parallelogram

go to slidego to slide

FAQs on Angles of a Parallelogram

Exercise Angles in a Parallelogram add up to 360°?

Yep, all the interior angles of a parallelogram add upwardly to 360°. For example, in a parallelogram ABCD, ∠A + ∠B + ∠C + ∠D = 360°. According to the angle sum holding of polygons, the sum of the interior angles in a polygon can be calculated with the help of the number of triangles that can be formed inside it. In this case, a parallelogram consists of 2 triangles, so, the sum of the interior angles is 360°. This tin also be calculated past the formula, S = (n − 2) × 180°, where 'n' represents the number of sides in the polygon. Here, 'n' = 4. Therefore, the sum of the interior angles of a parallelogram = S = (4 − 2) × 180° = (4 − 2) × 180° = two × 180° = 360°.

What is the Relationship Between the Adjacent Angles of a Parallelogram?

The adjacent angles of a parallelogram are besides known every bit sequent angles and they are always supplementary (180°).

How are the Reverse Angles of a Parallelogram Related?

The reverse angles of a parallelogram are ever equal, whereas, the adjacent angles of a parallelogram are always supplementary.

How to Find the Missing Angles of a Parallelogram?

We tin can easily find the missing angles of a parallelogram with the assistance of iii special properties:

• The opposite angles of a parallelogram are coinciding.
• The sequent angles of a parallelogram are supplementary.
• The sum of all the angles of a parallelogram is equal to 360°.

What are the Interior Angles of a Parallelogram?

The angles made on the within of a parallelogram and formed past each pair of adjacent sides are its interior angles. The interior angles of a parallelogram sum upwards to 360° and any two adjacent (consecutive) angles of a parallelogram are supplementary.

Are all Angles in a Parallelogram Equal?

No, all the angles of a parallelogram are not equal. There are two basic theorems related to the angles of a parallelogram which state that the opposite angles of a parallelogram are equal and the consecutive (adjacent) angles are supplementary.

What is the Sum of the Interior Angles of a Parallelogram?

The sum of the interior angles of a parallelogram is ever 360°. According to the angle sum property of polygons, the sum of the interior angles of a polygon tin can be institute by the formula, S = (n − 2) × 180°, where 'north' shows the number of sides in the polygon. In this example, 'n' = iv. Therefore, the sum of the interior angles of a parallelogram = Southward = (4 − 2) × 180° = (iv − 2) × 180° = 2 × 180° = 360°.

Are the Angles of a Parallelogram 90 Degrees?

In some parallelograms like rectangles and squares, all the angles measure xc°. However, the angles in the other parallelograms may not necessarily exist xc°.

Are the Reverse Angles of a Parallelogram Congruent?

Yes, the reverse angles of a parallelogram are coinciding. However, the adjacent angles of a parallelogram are always supplementary.

Are Consecutive Angles of a Parallelogram Congruent?

No, the consecutive (adjacent) angles of a parallelogram are not congruent, they are supplementary.

Are the Opposite Angles of a Parallelogram Supplementary?

No, co-ordinate to the theorems based on the angles of a parallelogram, the opposite angles are not supplementary, they are equal.

Source: https://www.cuemath.com/geometry/angles-of-a-parallelogram/

Posted by: hernandezwinger.blogspot.com

Share this post